Graduate programs in physics (and other science and engineering disciplines) often have some kind of exam that students have to take on the path to doctoral candidacy. Every place is a bit different. When I was an undergrad there, Princeton had a two-tiered exam system, with "prelims" largely on advanced undergrad level material and "generals" on grad-course-level content. Rice has an oral candidacy exam with subfield-specific expectations laid out in our graduate handbook. Stanford, when I went there in fall of 1993, had a written "qual", two days, six hours each day, ostensibly on advanced undergrad level material.
There are a couple of main reasons for exams like this: (1) Assessment, so that students learn the areas where they need to improve their depth of knowledge; (2) Synthesis - there are very few times in your scientific career when you really have to sit down and look holistically at the discipline. Students really do learn in preparing for such exams.
I've written about this particular exam experience here. Thanks to an old friend whose handwriting decorates some of the pages, here (pdf) is a copy of that exam (without the solutions). Wow. Brings flashbacks.
A blog about condensed matter and nanoscale physics. Why should high energy and astro folks have all the fun?
Search This Blog
Monday, April 29, 2019
Wednesday, April 24, 2019
Liquid droplets with facets
One essential concept in condensed matter physics is spontaneous symmetry breaking - the idea that the collective response of many components acting together results in a situation that has less symmetry than the underlying system. Crystalline solids are a classic example. The universe itself has (to high precision) "continuous translational symmetry" - the laws of physics governing some isolated system are the same as the laws of physics if you slide that system over a bit. Space has continuous rotational symmetry - reorienting your isolated system doesn't change anything. A collection of atoms, though, can assemble into a crystal, and the crystal structure itself has lower symmetry. For, e.g., an electron within the crystal, there is now discrete translational symmetry, meaning that the electron's environment is the same if the crystal is shifted not by arbitrary amounts, but by precise multiples of the lattice spacing of the crystal. Similarly, only specific discrete rotations of the lattice about particular axes leave the electron's environment unchanged.
We tend to think of liquids as not breaking continuous rotational or translational symmetry. If you consider timescales long compared to the jostling motion of atoms or molecules in a liquid, all positions look about the same, as do all directions in space. (It is possible to have intermediate situations, with liquids made from non-spherical molecules, and these can have directionality and local clustering. Such liquid crystals are used in the display you're most likely using to read this.) The lack of translational and rotational symmetry breaking in liquids is one reason that droplets (and bubbles) tend to be spherical. If it is energetically expensive to have an interface between, say, oil in water, for a fixed volume of oil, then the lowest energy situation is to have a spherical oil droplet - that minimizes the surface area of interface.
Yesterday I stumbled upon this paper, and that sent me down a literature rabbit-hole. This Nature paper (archived version here) led me to this PNAS paper, where I grabbed Fig. 1 at right. It turns out that it is possible to form faceted liquid droplets of certain oils (alkanes) in aqueous suspensions. The outermost layer of the alkanes acts rather like a lipid membrane, and it is possible to sit at a temperature where that layer crystallizes (the molecules in it spontaneously break translational and rotational symmetry) while the bulk of the droplet remains a liquid. What picks out the orientation of the resulting faceted shape? Spontaneous symmetry breaking. Tiny fluctuations or attributes of the local environment. Wild!
We tend to think of liquids as not breaking continuous rotational or translational symmetry. If you consider timescales long compared to the jostling motion of atoms or molecules in a liquid, all positions look about the same, as do all directions in space. (It is possible to have intermediate situations, with liquids made from non-spherical molecules, and these can have directionality and local clustering. Such liquid crystals are used in the display you're most likely using to read this.) The lack of translational and rotational symmetry breaking in liquids is one reason that droplets (and bubbles) tend to be spherical. If it is energetically expensive to have an interface between, say, oil in water, for a fixed volume of oil, then the lowest energy situation is to have a spherical oil droplet - that minimizes the surface area of interface.
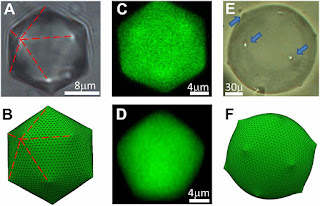 |
| Fig. 1 from Guttman et al., PNAS 113, 493-496 (2016). Faceted oil droplets! |
Yesterday I stumbled upon this paper, and that sent me down a literature rabbit-hole. This Nature paper (archived version here) led me to this PNAS paper, where I grabbed Fig. 1 at right. It turns out that it is possible to form faceted liquid droplets of certain oils (alkanes) in aqueous suspensions. The outermost layer of the alkanes acts rather like a lipid membrane, and it is possible to sit at a temperature where that layer crystallizes (the molecules in it spontaneously break translational and rotational symmetry) while the bulk of the droplet remains a liquid. What picks out the orientation of the resulting faceted shape? Spontaneous symmetry breaking. Tiny fluctuations or attributes of the local environment. Wild!
Wednesday, April 17, 2019
Brief items, + "grant integrity"
As I have been short on time to do as much writing of my own as I would like, here are links to some good, fun articles:
- Ryan Mandelbaum at Gizmodo has a very good, lengthy article about the quest for high temperature superconductivity in hydrogen-rich materials.
- Natalie Wolchover at Quanta has a neat piece about the physics of synchronization.
- Adam Mann in Nat Geo has a brief piece pointing toward this PNAS paper arguing the existence of a really weird state of matter for potassium under certain conditions. Basically the structure consists of a comparatively well-defined framework of potassium with 1D channels, and the channels are filled with (and leak in 3D) liquid-like mobile potassium atoms. Weird.
Tuesday, April 16, 2019
This week in the arxiv
A fun paper jumped out at me from last night's batch of preprints on the condensed matter arxiv.
arXiv:1904.06409 - Ivashtenko et al., Origami launcher
A contest at the International Physics Tournament asked participants to compete to see who could launch a standard ping-pong ball the highest using a launcher made from a single A4 sheet of paper (with folds). The authors do a fun physics analysis of candidate folded structures. At first, they show that one can use idealized continuum elasticity to come up with a model that really does not work well at all, in large part because the act of creasing the paper (a layered fibrous composite) alters its mechanical properties quite a bit. They then perform an analysis based on a dissipative mechanical model of a folded crease matched with experimental studies, and are able to do a much better job at predicting how a particular scheme performs in experiments. Definitely fun.
There were other interesting papers this week as well, but I need to look more carefully at them.
arXiv:1904.06409 - Ivashtenko et al., Origami launcher
A contest at the International Physics Tournament asked participants to compete to see who could launch a standard ping-pong ball the highest using a launcher made from a single A4 sheet of paper (with folds). The authors do a fun physics analysis of candidate folded structures. At first, they show that one can use idealized continuum elasticity to come up with a model that really does not work well at all, in large part because the act of creasing the paper (a layered fibrous composite) alters its mechanical properties quite a bit. They then perform an analysis based on a dissipative mechanical model of a folded crease matched with experimental studies, and are able to do a much better job at predicting how a particular scheme performs in experiments. Definitely fun.
There were other interesting papers this week as well, but I need to look more carefully at them.
Monday, April 08, 2019
Brief items
A few brief items as I get ready to write some more about several issues:
- The NY Times posted this great video about using patterned hydrophobic/hydrophilic surfaces to get bouncing water droplets to spin. Science has their own video, and the paper itself is here.
- Back in January Scientific American had this post regarding graduate student mental health. This is a very serious, complex issue, thankfully receiving increased attention.
- The new Dark Energy Spectroscopic Instrument has had "first light."
- Later this week the Event Horizon Telescope will be releasing its first images of the supermassive black hole at the galactic center.
- SpaceX is getting ready to launch a Falcon Heavy carrying a big communications satellite. The landing for these things is pretty science-fiction-like!
Tuesday, April 02, 2019
The physics of vision
We had another great colloquium last week, this one by Stephanie Palmer of the University of Chicago. One aspect of her research looks at the way visual information is processed. In particular, not all of the visual information that hits your retina is actually passed along to your brain. In that sense, your retina is doing a kind of image compression.
Your retina and brain are actually anticipating, effectively extrapolating the predictable parts of motion. This makes sense - it takes around 50 ms for the neurons in your retina to spike in response to a visual stimulus like a flash of light. That kind of delay would make it nearly impossible to do things like catch a hard-thrown ball or return a tennis serve. You are able to do these things because your brain is telling you ahead of time where some predictably moving object should be. A great demonstration of this is here. It looks like the flashing radial lines are lagging behind the rotating "second hand", but they're not. Instead, your brain is telling you predictive information about where the second hand should be.
People are able to do instrumented measurements of retinal tissue, looking at the firing of individual neurons in response to computer-directed visual stimuli. Your retina has evolved both to do the anticipation, and to do a very efficient job of passing along the predictable part of visualized motion while not bothering to pass along much noise that might be on top of this. Here is a paper that talks about how one can demonstrate this quantitatively, and here (sorry - can't find a non-pay version) is an analysis about how optimized the compression is at tossing noise and keeping predictive power. Very cool stuff.
Your retina and brain are actually anticipating, effectively extrapolating the predictable parts of motion. This makes sense - it takes around 50 ms for the neurons in your retina to spike in response to a visual stimulus like a flash of light. That kind of delay would make it nearly impossible to do things like catch a hard-thrown ball or return a tennis serve. You are able to do these things because your brain is telling you ahead of time where some predictably moving object should be. A great demonstration of this is here. It looks like the flashing radial lines are lagging behind the rotating "second hand", but they're not. Instead, your brain is telling you predictive information about where the second hand should be.
People are able to do instrumented measurements of retinal tissue, looking at the firing of individual neurons in response to computer-directed visual stimuli. Your retina has evolved both to do the anticipation, and to do a very efficient job of passing along the predictable part of visualized motion while not bothering to pass along much noise that might be on top of this. Here is a paper that talks about how one can demonstrate this quantitatively, and here (sorry - can't find a non-pay version) is an analysis about how optimized the compression is at tossing noise and keeping predictive power. Very cool stuff.
Subscribe to:
Comments (Atom)